“Show your work.” Have you ever tried to ask a group of math teachers what this actually looks like? Answers can vary, but in general, even the teachers who say this often have a hard time describing what “good showing work” is. While this can be frustrating, going back to the first principles of “why” can help us figure out the “how.” (To read about some of those reasons “why”, check out Why to Encourage Your Child to Show Their Thinking.)
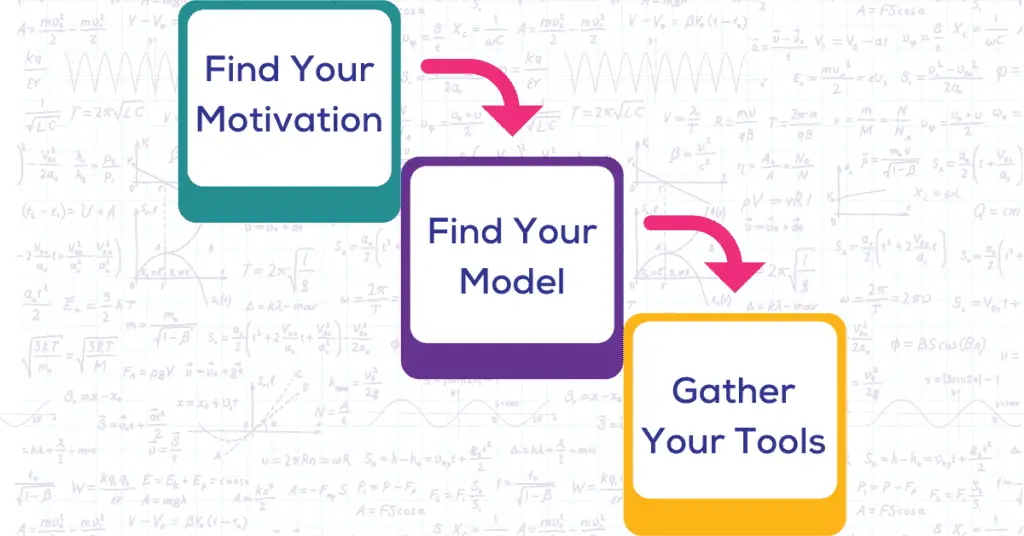
To determine how to show work, and how much work to show, think about why. Options include following the steps of an example, showing your own thought process and solution skills, and practicing a balance of summarizing vs details.
Extrinsic reasons for showing work, such as the teacher’s wanting to know whether you’ve mastered a certain procedure, means showing the work as it was demonstrated in class. In these cases, your best bet is to consider an example and follow the steps and the setup of the example. Intrinsic reasons, such as wanting to practice and learn to communicate your own thoughts or show your own brilliance, means showing your own thought process and solution skills, after distilling out the important steps and finding a way to balance summarizing your process versus telling enough to help your reader understand.
This latter process forms the basis of most of education, and math education in particular. Specifically, humanity has gathered millennia of knowledge, mostly from trial and error. Education is built by the process of judging which of those pieces of knowledge are important, summarizing the process and discoveries, and passing them along as prepackaged information to the next generations. It’s normal for educators to disagree about any of these judgments and summaries, just as it would be normal for different people to choose different priorities and ways of summarizing their own arguments and strategies. Be patient with yourself and your child, and use feedback in context to revise how you communicate your own summaries.
A ‘good’ answer is one where I can follow their thoughts/logic.
biology professor Samantha elliott
How do you show work in math?
The first goal of showing your work, no matter the motivation, is to communicate the process. Whether that process is a specific model demonstrated in a textbook or in class, or whether that process is your own, the goal is to balance showing enough detail that someone else can follow the reasoning, but also to leave out distracting details that would simply bore the reader and lead them to “tune out.” Thus, organizing the work to show the flow of one step to another is often important. Writing phrases and sentences, as well as labels, is helpful. Working out the problem on scratch paper, then copying over the important steps to the page that will be turned in is a helpful practice to build the skill of summarizing. This can take more time at first, and is hard to convince our children it’s worth it. However, remember, this is how knowledge is passed down from generation to generation. Only some lessons are left to be learned by discovery, and we constantly try to summarize and share the lessons we learned in order to save our children’s time and disappointment.
In practicalities, some things to try:
- Look for an example and ask your child to follow that example but with the numbers given in the new problem.
- Ask your child to explain what they did in words, and help them to write those same words on the paper.
- Ask them if there are any pictures that would be useful to draw.
- Ask your child what they are picturing in their head when they “solve” this problem. Can they draw that on paper?
- After they put some ideas on paper, ask them what order those ideas should be in. Are they presented in that same order?
- “Read” or explain their work out loud back to them. Try to stick to only and exactly what they’ve shown on the paper. Then ask them “did I get everything?”
- Play “school.” You are the student and your child is the teacher. Have a worksheet that your child has to “grade” and help you improve on. (Consider using a topic your child really knows well.) Sometimes show your work. Sometimes don’t. Ask your child which was more helpful for them to know how to help you.
- Talk to your children in small conversations throughout the day, about situations where you or where their teachers summarize knowledge to help them or others understand something that it took a long time to learn. In other words, normalize that process of learning and of passing along knowledge.
- Help your child learn to draw lines when they need, to label steps when they need, to use grids or technology appropriately, and in general to put tools in place that help them organize their thoughts for effective communication. This is not to force them to conform to a normalized standard (though some teachers will indeed ask this), but rather to help them build just enough organizational skills, tailored to their own needs, to be effective communicators.
How can students show math work digitally?
Technology has made astonishing advances in communicating mathematics. Most likely, once they get to middle school, high school, and even college, your children will be doing their homework online, submitting answers there, and getting instant feedback. This can actually make it harder to convince them to show their work. Yet, their teachers likely will still demand they show their thinking on exams and in-class quizzes and activities. You might find value in practicing the work in the same way it’ll be asked for on these exams, etc. If you need to show work digitally, here are a few ideas:
- Show handwritten work on paper. Then take a *clear* picture with your phone or scanner and submit the image.
- Write/type sentences describing the process used.
- Make a quick sketch of any graphs created by technology, labelling the important points or aspects used to answer the questions. (Then take a picture and submit the picture if needed.)
- Take and submit a screenshot. If possible, write on the screenshot with arrows or labels describing the pieces actually used to answer the question.
- Use an online whiteboard (there are many!) or other tool such as polypad.org that has a whiteboard component, then take a screenshot.
What if my student is neurodivergent or has physical or fine-motor limitations?
It’s impossible to list all possible adaptations, but here are a few to get your creativity going. Then discuss with your child’s teacher to figure out what will work for all of you.
- Video or audio record your child describing their answer verbally (likely to work best for the teacher if they actively use google classroom or another learning management system that can sort individual student work for them)
- Provide graph or grid paper to your child.
- Take advantage of the progress in math typography to use tools such as polypad.org or word processing equation editors with shallow learning curves.
- Use tools such as the number cards at polypad.org or physical number and symbol cards with a camera to allow students to drag-and-drop to show their work.
- Invest in a vertical whiteboard/chalkboard easel and thick-barreled markers or chalk. Then take and submit pictures of the work the child does. (This is also the type of thing that is frequently gifted and received in my local Buy Nothing group if you’re looking for a cost-free version.)
- Use a label-maker, such as shown in the article A Label Maker Can Be Assistive Technology for Worksheets on the Least Restrictive Learning blog.
What other ideas do you have that should be on these lists? Let’s be each other’s village as we learn to help our children the best way we can.
You’ve Got This!
